A unique feature of the CMP curriculum is that curriculum-generated student work is a context for student learning of mathematics. This project focuses on the role of student work found in curriculum materials and its potential for improving the teaching and learning of mathematics (Edson et al., 2017; Gilbertson et al., 2016; Gilbertson et al., 2016). In CMP4, the use of student work found in the Student Editions and teacher support help to (1) enhance student and teacher conceptual understanding and procedural fluency; (2) develop student agency, identity, authority, and ownership of mathematics; and (3) broaden student and teacher capacity to make sense of situations.

Over the years our knowledge of how and what students understand and the role of the teacher in this process has grown exponentially, Over the years the problems have taken on the burden of sharing this knowledge. For CMP4 we had the chance to deeply reflect on what is the challenge being presented; what is the embedded mathematics in the challenge; and how is this new knowledge connected to prior and future knowledge. Thus the STEM problem format consists of three phases: Initial Challenge, What If . . . ? and Now

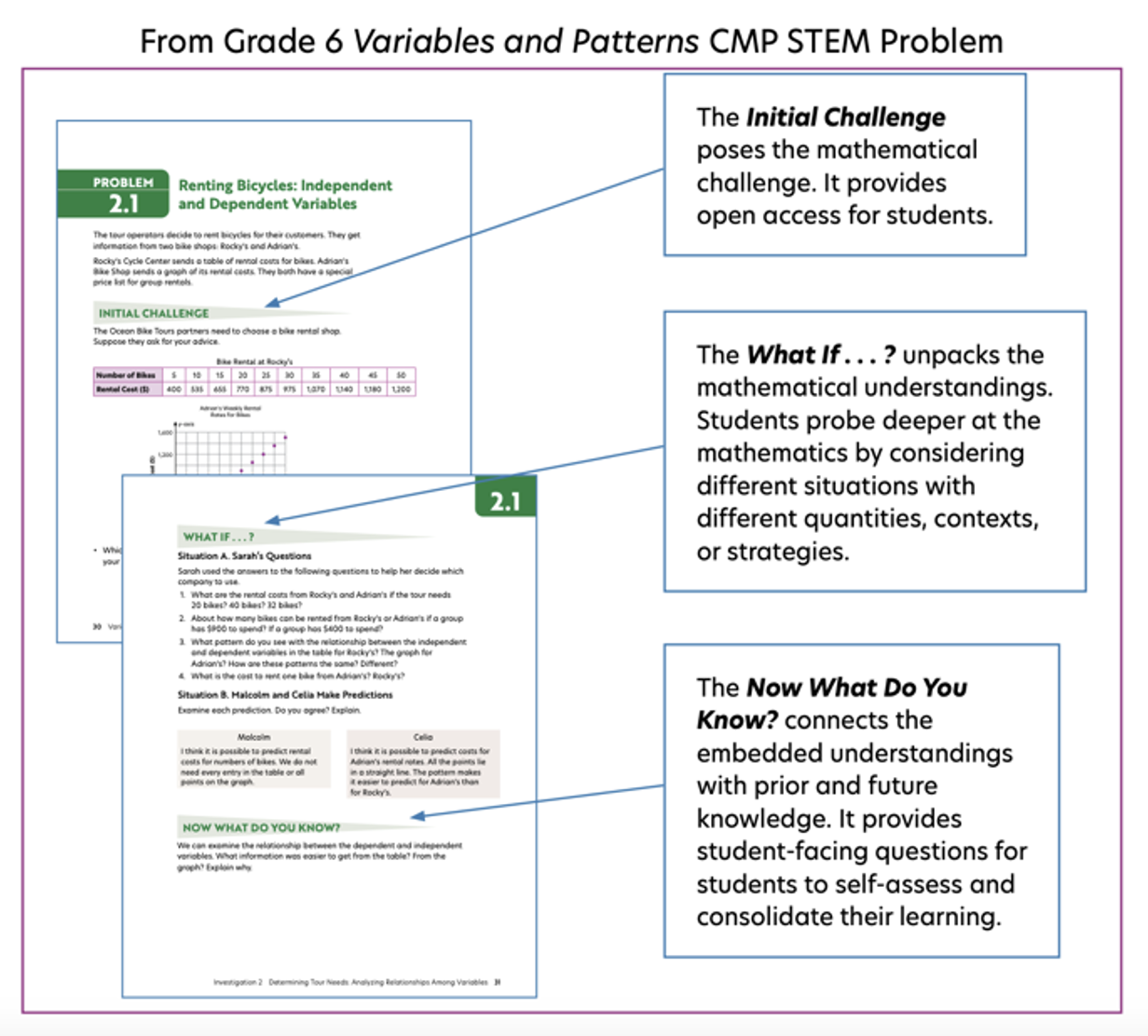
The Arc of Learning^TM Framework is a resource for curriculum design and use that makes explicit the intentions of the curriculum designers about how students engage in the learning of mathematics over time (Edson et al., 2016, 2017a, 2017b, 2019; Edson et al., 2019; Edson et al., 2015).

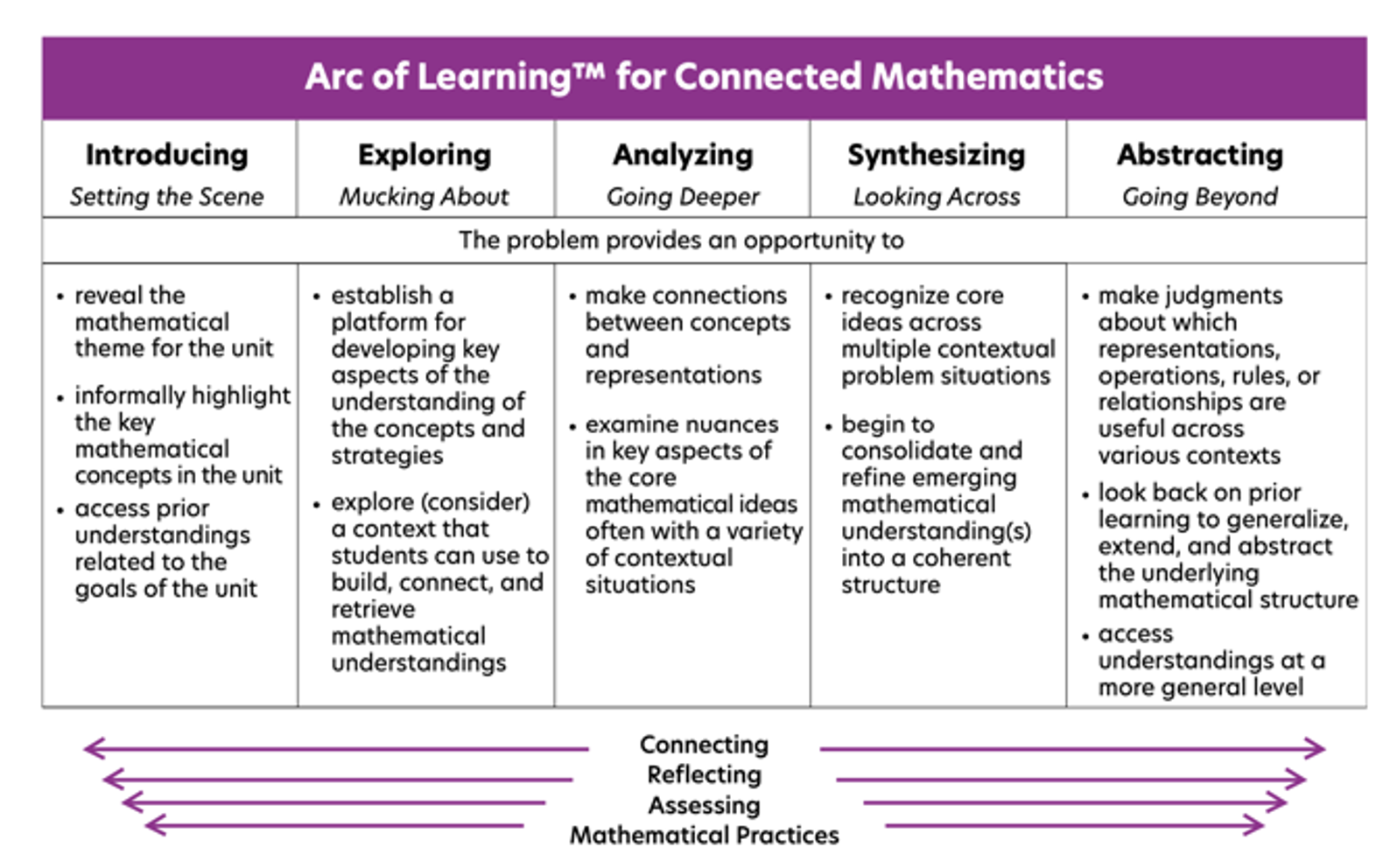
In CMP4, the Arc of Learning^TM framework informs

• how student thinking and learning is targeted and how that thinking and learning might unfold within and across mathematics sequences of problems;
• how teachers understand the development of long-term mathematical goals embedded in a coherent sequence of problems;
• the mathematical, pedagogical, and assessment decisions teachers make when planning or enacting lessons that respond to students’ mathematical conceptions;
• professional development to support problem-based learning; and the research and design on problem-based, contextualized curriculum.

As learners need different supports at different times in their learning, teachers attend to these diverse learning needs throughout classroom instruction. In CMP4, the Attending to Individual Learning Needs Framework provides teachers with ways to support diverse learning needs in rich mathematics problem-solving environments (Edson & Slanger-Grant, 2024, 2021; Edson et al., 2023). The differentiation framework highlights dimensions needed to enhance how students can access and engage with deep mathematical learning. The framework supports teachers in developing students as knowers and doers of mathematics by focusing on students’ strengths, backgrounds, and experiences. The five components of the framework include

• establishing a problem-solving environment that advances the mathematical understandings for each student;
• developing student agency, identity, and ownership of the mathematics;
• enhancing the portrayal of the mathematical ideas;
• bridging language to connect with students’ mathematical understandings; and
• allowing sufficient time to learn mathematical ideas through a sequenced set of tasks.

The teacher support materials give teachers guidance on using the Attending to Individual needs components.

Teachers assess student learning of mathematics throughout a CMP lesson. This project focuses on developing a set of resources to help mathematics teachers and instructional leaders make sense of formative assessment as an ongoing process in CMP classrooms. (Ray et al., 2017; Ray et al., 2016; Ray et al., 2016; Ray et al., 2015). This project was funded by CMP royalties from the MSU authors and administration to support research and development in mathematics education.

In this project, we report on field-testing and professional learning experiences of the fourth edition of the Connected Mathematics curriculum involving over 500 teachers from the United States and six countries. We ground our study in the theoretical and empirical curriculum design and enactment tensions that emerge in problem-based mathematics classrooms. We report on field-testing and professional learning experiences of the fourth edition of the curriculum involving over 500 teachers from the United States and six countries. This includes data analysis and feedback from four years of field-testing that reflect the interactions between teachers and students with the materials, including unit and problem feedback, teacher support, and assessment feedback to highlight changes made in curriculum design. Finally, we discuss implications for how the curriculum design and development process helps provide cohesive and effectively sequenced materials to support students and teachers in rich mathematical problem-solving experiences. (2025 paper in progress.)

This project is designing course materials for preservice teachers (PST) using some part of Connected Mathematics4. The materials provide four modules, each of which consists of four or five lessons. Each lesson contains two-part activities: (Part 1) PSTs experience student and teacher roles in solving mathematics problems embedded within real-world contexts), and (Part 2) PSTs reflect on teacher roles in such student-centered learning environments to connect with research-based teaching practices, theories, and pedagogy in mathematics education literature. The Part 1 activity utilizes one CMP curriculum unit (Moving Straight Ahead) that includes both the Student Edition and the Teacher Guide. Materials for each lesson include learning goals for PSTs, lesson outlines, suggested reading assignments, suggested discussion questions, additional readings/resources, and handouts. The modules aim to support PSTs in developing a deeper understanding of teaching and learning mathematics in a problem-based, inquiry-oriented classroom. Each of the four modules has both mathematical and pedagogical themes. (2024-in progress).

The royalties that MSU receives from the sales of its existing editions of CMP are used to fund research and development in mathematics and science education, including continual evaluation and revision of the CMP curriculum. These funds are also used to build capacity for mathematics educators and educational researchers. This includes several Lappan- Phillips Endowed Professorships and a Lappan-Phillips-Fitzgerald Endowed Mathematics Education Chair at Michigan State University. So far, over 70 graduate students have received assistantships, with many of them completing their dissertations in CMP classrooms; the funds have also supported science educators and postdoctoral researchers. These funds also provide professional development and leadership opportunities for teachers and district leaders. Out of the 20,000 participants at CMP events, more than 300 CMP ambassadors have served as workshop leaders, conference presenters, and mathematical coaches under the mentorship of the CMP author team.

A unique feature of the CMP curriculum is that curriculum-generated student work is a context for student learning of mathematics. This project focuses on the role of student work found in curriculum materials and its potential for improving the teaching and learning of mathematics.

Supporting rich student learning, discourse, and collaboration in technology-enhanced classrooms can be a significant challenge as technology can act as an impediment to conversation. This project supports the development of a collaborative digital learning environment that embeds rich middle school mathematics tasks. The project aims to understand how students' individual and collaborative engagement in learning mathematics is enhanced by the digital platform, and how student engagement and learning is affected over the course of a year-long seventh grade course. The project will develop the initial digital collaborative platform using the Connected Mathematics Project materials, a rich, problem-based middle grades mathematics curriculum. Using digital notebooks, platform analytics, and other class artifacts, the team will develop a set of design characteristics for digital platforms that support student collaboration in learning mathematics.

This project will explore two key questions related to student learning and collaboration in the technological platform: how does the platform enhance students' individual and collaborative engagement, and how do student learning outcomes, engagement, and attitudes develop over an academic year in which the platform is used repeatedly. The project will use the platform to collect data related to student collaboration, engagement, instructional practices, classroom artifacts, and written and spoken mathematical reflections from students and teachers. The research outcomes will include guidelines for a research-based approach to the use of learning analytics in technology-enhanced mathematics education, an understanding of design features that support meaningful STEM learning using digital notebooks, and the nature of the teacher-student boundaries in using digital learning resources to support students' individual needs. The project will focus on a deep examination of a key mathematical idea in the seventh-grade curriculum, proportional reasoning, to understand how students' understandings are influenced over time using the platform. Project activities will include development and testing of the platform with students, pilot and field testing with teachers, and professional learning opportunities for teachers to ensure thoughtful implementation of the platform in practice.

The project will create a digital environment for middle school mathematics teachers to promote collaboration. The digital environment for the teachers links to a student collaborative environment and contains the same problem-based curriculum materials. The environment helps teachers to collaborate and learn from one another. This occurs when teachers plan, teach, and reflect on student learning. The online, digital platform will help teachers work together more easily in networks that might be at different schools. The resources online will include problem-based curriculum materials, classroom artifacts from students, and resources created by teachers. The project will learn about how teachers use resources, collaborate in the digital environment, and support each other through the network. With more curriculum resources being created for online teaching and learning, the project will help understand how mathematics teaching and learning can be best supported.

The design-based research project in mathematics studies a digital, collaborative environment for teachers that is combined with a student collaborative platform for a middle school problem-solving curriculum. The goal is to design and develop the digital collaborative platform so networks of teachers can create, use, and share teaching resources for planning, enactment, and reflection on student thinking. The project will include middle school teachers in the design process. The environment for students enables student thinking to be visible to other students and the teacher. This allows the teacher to archive and then examine student reasoning and thinking. The new environment will allow that work to be shared with other teachers in a professional learning community. The research question is: how do teachers in networks access, generate, use, and share teaching resources (including classroom artifacts) as needed to support mathematics teaching (planning, enactment, and reflection of student thinking)? The project will use teacher interviews and artifacts from the collaborative environment for mixed methods data collection and analysis. Teacher reflection resources will be used to understand how they are thinking about students? mathematical work and how they are using the platform resources. 

The study will contribute knowledge about how to support students' learning and engagement in mathematics using a digital curriculum platform. The goal of the project is to study how the use of open-ended problems and "just-in-time" supports in a digital platform can promote inquiry-based mathematics learning. Increasing numbers of schools are using digital curriculum for mathematics. "Just-in-time" supports will give the students options for seeking help as they are working on an open problem or give teachers the opportunity to generate prompts for students working in small groups as needed. The digital environment provides an opportunity for small groups of students to work on challenging tasks and decide when they need help. The project is building on a widely adopted middle school mathematics curriculum and tasks that are being used in a range of settings. The project will examine middle school students' mathematics learning and productive disciplinary engagement as well as teachers' role in the classroom environment. This project is supported by NSF's EHR Core Research (ECR) program. The ECR program emphasizes fundamental STEM education research that generates foundational knowledge in the field.

The research will investigate three questions. (1) How can productive disciplinary engagement be fostered in digital learning environments with open problems and "just-in-time" supports? How can student learning of mathematics be enhanced? (2) What is the nature of productive disciplinary engagement and student learning of mathematics at key development points in a connected sequence of problems and lesson goals? (3) What information do teachers draw upon when they use open problems and "just-in-time" supports? How do teachers adapt the supports for specific problems? It is situated in a design-based research framework for research relying on multiple qualitative and quantitative sources of data. The project will go through three cycles of design: prototype testing with students to investigate productive disciplinary engagement as a construct, classroom-based pilot testing to understand the teacher's role, and broader field testing to extend knowledge of how students' use open problems and "just-in-time" supports. The analysis of students' data will document how they problematize mathematics situations, how ideas are developed in a small group via students' positioning themselves in the conversation, and how students use disciplinary norms and arguments for their ideas. Students' interactions with the tool, particularly the "just-in-time" supports will be incorporated to understand how ideas develop. Assessments will be used to document students' learning of the mathematics. The teachers' role will be analyzed via classroom video, interviews, and other artifacts of instruction.

The primary goal of this project is to help middle school students deepen and communicate their understanding of mathematics. The project will develop and test a digital platform for middle school mathematics classrooms. The digital platform will allow students to collaboratively create representations of their mathematics thinking, incorporate ideas from other students, and share their work with the class. The digital learning environment makes use of a problem-centered mathematics curriculum that evolved from extensive development, field-testing and evaluation, and is widely used in middle schools. The research will also contribute to understanding about the design and innovative use of digital resources and collaboration in classrooms as an increasing number of schools are drawing on these kinds of tools. Projects in the DRK-12 program build on fundamental research in STEM education and prior research and development efforts that provide theoretical and empirical justification for proposed projects.

The project will support students to collaboratively construct, manipulate, and interpret shared representations of mathematics using digital inscriptional resources. The research activities will significantly enhance our understanding of student learning in mathematics in three important ways. The project will report on how (1) evidence of student thinking is made visible through the use of digital inscriptional resources, (2) student inscriptions are documented, discussed, and manipulated in collaborative settings, and (3) students' conceptual growth of big mathematical ideas grows over time. An iterative design research process will incorporate four phases of development, testing and revision, and will be conducted to study student use of the digital learning space and related inscriptional resources. Data sources will include: classroom observations and artifacts, student and teacher interviews and surveys, student assessment data, and analytics from the digital platform. The process will include close collaboration with teachers to understand the implementation and create revisions to the resources.

CMP started with the NSF-funded Middle Grades Mathematics Project (MGMP) which produced 5 exemplary middle school mathematics unit. Each problem-based unit was designed to develop students’ understanding of an important mathematical idea. It also introduced the Launch-Explore-Summarize (LES) inquiry-based classroom.
1984-86:19NSF/SED-80-18025

Several professional learning grants supported the implementation of the MGMP units.

• 1984: NSF/DPE-83-17063
• 1986: NSF/TEI-86-51686
• 1988-91: NSF/TPE-88-50505
• The Teaching Algebra Project
  • 1986-89. NSF/TEI-85-51686

• 1991-1996: NSF/ESI-93-55542
• 1993-96  NSF funded Research Supplement to the Connected Mathematics Project
• 1995-98. NSF/ESI-93-55542 Connecting Teaching, Learning, and Assessment

NSF/ESI-9986372

CMP with NSF funding partnered with the ShowME Center at the University of Missouri to disseminate information on the five NSF-funded middle mathematics curriculum.

1997–02: NSF/ESI-97-14999

2002-06: NSF/CG004954-3

CMP partnered with the University of Missouri, University of Chicago, and Western Michigan University to student mathematics curricula.

2004-14: NSF/ESI-0333879   

CMP3 was funded by Michigan State Universities royalties from CMP1 and CMP2.

 CMP4 was funded through Michigan State University’s royalties from CMP1, CMP2, and CMP3.