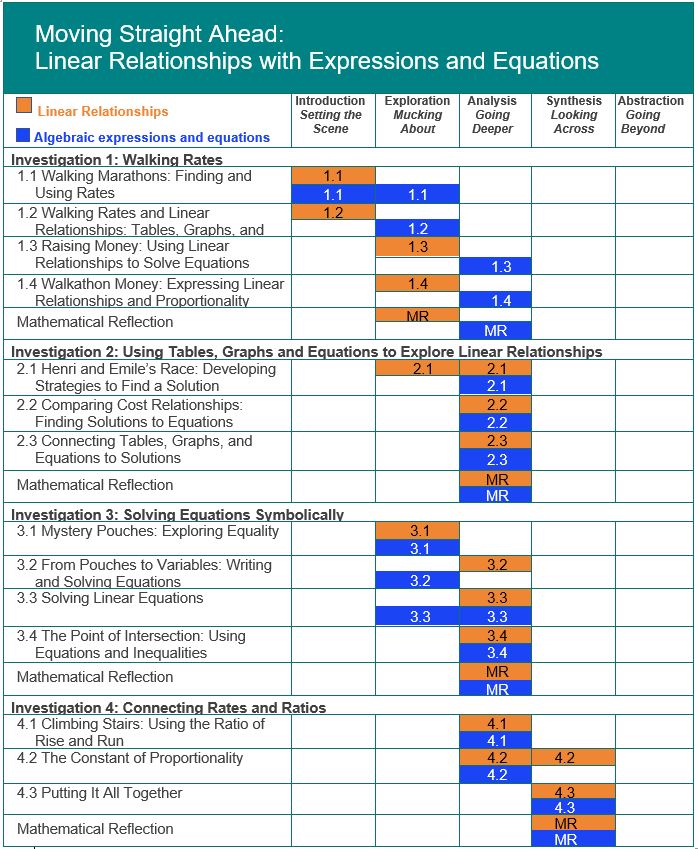
Moving Straight Ahead
Revision Comments
The CMP4 MSA unit has 14 problems compared to the 17 problems for CMP3 MSA.
This unit continues the exploration of analyzing and representing quantitative relationships between independent and dependent variables that was started in the 6th grade Variables and Patterns unit. This unit continues the exploration by using linear relationships as a context to explore what it means to be linear, but also to strengthen students’ use of linear expressions, linear equations and linear inequalities. It also continues to strengthen students’ understanding of proportional reasoning which was explored in several previous 6th and 7th grade units including Comparing Quantities, Stretching and Shrinking, and Comparing and Scaling.
Since CMP is a contextual problem-based curriculum, starting with contextual situations naturally allows for two variables to surface which in turn motivates ways to express these relationships using tables, graphs, expressions and equations in addition to verbal representations. This unit keeps the study of relationships on the table at the same time it deepens students’ understanding of linear expressions, equations y = ax + b, and inequalities ax +b < c or ax + b > c. Throughout the unit students seek information about one variable given the value of the other variable. This can be done by reasoning numerically, using tables or graphs, or symbols. Expressions and equations surface as a way to express linear relationships. Thus, the contextual situation provides students with a way to write, interpret, and manipulate symbolic linear expressions, equations and inequalities. Contextual situations also provide students with a context to develop and retrieve understanding of linearity and at the same time develop understanding of linear symbolic expressions and equations.
The first Investigation starts with the walking rate experiment and is similar to CMP3 with some changes to Investigation and Problem names to reflect use of linear expressions and linear equations and that linear equations can be solved with tables and graphs. Investigation 2 is similar to CMP3 with more emphasis on the relationship between data points in a table and graph to solutions of linear equations, y = ax +b. Investigation 3 is similar to CMP3 using pouches and coins to develop properties of equality for solving linear equations. Investigation 4 has the biggest changes. Slope is introduced as a way to express the linear rate of change patterns and thus connect slope as ratio to rate of change. Slope is introduced as the ratio, a vertical change for every one unit of horizontal change. It is connected to linear rate of change patterns observed in the table. The ratio of vertical change to horizontal change is equal no matter where it is measured on a line. All the ratios (rise: run) are equal to rate of change or coefficient of x (or constant of proportionality, if it is also a proportional relationship.)
The following set of questions guide the development of all of the CMP algebra units. In future units, the study of linear relationship resurface as they are compared with inverse relationship, exponential, quadratic, polynomial, or rational relationships.
- What are the variables in the problem?
- Do the variables in the problem have a linear relationship to each other? What patterns in the problem suggest that the relationship is linear? Proportional?
- How can the linear relationship in a situation be represented with a verbal description, a table, a graph, or an equation?
- How do changes in one variable affect changes in a related variable? How are these changes captured in a table, a graph, or an equation?
- How can tables, graphs, and equations of linear relationships be used to answer questions?
CMP STEM Problem Format
What is a CMP STEM Problem?
In CMP, the class discussions attend to three important features of the goal for a mathematics problem: (1) student strategies and problems solutions, (2) the embedded or encoded mathematics of the problem, and (3) connections to prior learning and future knowledge. To continue to support these discussions, the CMP problems have been newly designed to support STEM education. Rather than using conventional numbering and lettering (e.g., A1, A2, B1, B2, B3, etc.), the CMP problems now use three important components.
What are the components of a CMP STEM problem?
Problem
Initial Challenge
Component Description
The Initial Challenge contextualizes the problem and presents the challenge. The IC also provides an opportunity for open access to the mathematical challenge of the problem.
What If...?
The What If…? unpacks the embedded or encoded mathematics of the problem. The WI…? provide students with further opportunities to probe at the mathematics. Situations focus on what happens when you consider changed quantities or parameters, new aspects to the context, or returning to mathematical ideas mentioned earlier. Situations also focus on different solution strategies and work that students can do to solve the problem.
Now What Do You Know?
The Now What Do You Know connects learning to prior knowledge and consider future payoffs. As with the Focus Questions in the CMP3 Teacher’s Guide, the NWDYK provides focus on the learning goal of the mathematics problem. Whether students write answers is up to the teacher, but written and verbal discussions occur in the Problem Summary and in the Mathematical Reflections that span across problems.
Why do CMP STEM Problems matter?
Often STEM professionals work to solve problems and meaningfully connect these solutions to inform the needs of society. The complexities of the world today necessitate that each and every student be prepared with the knowledge and skills to solve difficult problems, gather and evaluate evidence, and make sense of information. To promote learning that resembles the work of STEM professionals, the problems in CMP are redesigned using the new format to help produce a population of students that is more reflective of the wider STEM community.
How does the Launch-Explore-Summary instructional model connect to the CMP STEM Problems?
While CMP STEM Problems contain Initial Challenge, What If…? and Now What Do You Know components, the instructional model of Launch/Explore/Summary continues to be critical for CMP STEM Problems. In some ways, the Initial Challenge can be thought of as the box questions in CMP3. In the Launch, all three components are launched. There may be an occasion where teachers will want to have a brief summary before students go to the What If…? component. These are indicated in the teacher materials. The Now What Do You Know is intended for students to reflect on and be prepared to talk about what they have learned in the Summary. A teacher may choose to have students record some of their understandings in a “learning log” at the end of the Summary. These may be useful when students complete the Mathematical Reflections.
How do the Mathematical Reflections connect to the CMP STEM Problems?
The Mathematical Reflections build on the Now What Do You Know of each problem in the unit. Rather than having a Mathematical Reflection with different questions for each Investigation, the Mathematical Reflections are now streamlined around one essential question. For example, the Mathematical Reflection for each Investigation in Stretching and Shrinking: Developing Proportional Reasoning in the Context of Similarity (Scale Drawings) is:
Mathematical Reflection
In this unit, we used proportional relationships to investigate similar figures or scale drawings, including how to determine if two figures are similar. In this Investigation,
What do you know about similarity? How were proportional relationships used to study similarity?
Unit Descriptions
This unit continues the exploration of analyzing and representing quantitative relationships between independent and dependent variables that was started in the 6th grade Variables and Patterns unit. This unit uses linear relationships as a context to explore what it means to be linear and to strengthen students’ use of linear expressions, linear equations, and linear inequalities.
Since CMP is a contextual problem-based curriculum, starting with contextual situations naturally allows for two variables to surface which in turn motivates ways to express these relationships using tables, graphs, expressions, and equations in addition to verbal representations. This unit keeps the study of relationships in the foreground at the same time it deepens students’ understanding of linear expressions, equations y = ax + b, and inequalities ax +b < c or ax + b > c. Throughout the unit students seek information about one variable given the value of the other variable. This can be done by reasoning numerically, using tables, graphs, or symbols. Expressions and equations surface as a way to express linear relationships. Thus, the contextual situation provides students with a way to write, interpret, and manipulate symbolic linear expressions, equations, and inequalities. Contextual situations also provide students with a context to develop and retrieve understanding of linearity and at the same time develop understanding of linear symbolic expressions and equations.
This idea of linearity is introduced in Investigation 1 with an experiment in which students determine their walking rates to prepare for a walkathon. This experiment is closely tied to the central idea of rate of change between two variables, and it provides the idea of “walking rate” within the walkathon theme for the unit.
While identifying, representing, and interpreting linear relationships is the central idea in this Unit, students also work with linear inequalities and equivalent expressions throughout the unit. Solving linear equations and writing equations for lines is also explored and will be revisited with more complexity in 8th grade Units—in particular, in Thinking with Mathematical Models and Say It With Symbols. The context of linearity also allows provides an opportunity for students to continue to develop deep understanding of proportional reasoning. In particular, they deepen their understanding of linear situations by exploring linear situations represented by y = ax, which is proportional, and linear situations represented by y = ax + b, where b ≠ 0 is not proportional.
As with all of the CMP4 units one Mathematical Reflection guides the development of the understanding of the mathematical ideas in the unit.
Mathematical Reflection
In this unit we are continuing to explore patterns of change between two variables using linear situations. We use tables, graphs, expressions, equations, and inequalities to represent and these linear patterns and solve problems. At the end of this Investigation,
What do you know about linear expressions, equations, inequalities, and relationships?
Summary of Investigations
Investigation 1: Walking Rates
The rates at which students walk and the amount of money per kilometer that sponsors donate for a walkathon are two contexts for this Investigation. Students look at the patterns of change for each relationship and the effect of those patterns on various representations. In Problem 1.1 they determine their own walking rate and represent it in a table and graph to determine how far they walk in a certain amount of time or the time it will take to walk a certain distance. They begin to recognize linearity as a straight line. In Problem 1.2 they use walking rates of three students to examine the pattern of change between the two variables (walking rate and distance walked) as represented in a table, graph, or equation of the relationship. In Problem 1.3 they use the context of pledge plans of their sponsors and explore whether this relationship is linear. In Problem 1.4 they determine if the relationship of the amount of money donated over time to charity by two classes is linear. They notice that the rate of change is negative and that the corresponding graphs that slant down from left to right are linear.
At the end of this Investigation students begin to see that as the independent variable changes by a consistent amount, there is a corresponding consistent change in the dependent variable.
For linear relationships, this pattern of change is a rate of change. At this point, some students will begin to recognize that rate of change is the coefficient of x in the general equation y = ax + b. They use tables, graphs, and numerical reasoning to solve linear equations and inequalities. They continue to explore which linear relationships are also proportional, an idea that has been developed over the course of multiple units in 6th grade and in Stretching and Shrinking and Comparing and Scaling in 7th grade.
Investigation 2: Using Tables, Graphs, and Equations to Explore Linear Relationships
This Investigation continues the theme of walkathons and helps students deepen their understanding of patterns of change. The rate of change between the two variables in a linear relationship and the y-intercept of the graph of a linear relationship are formalized in this Investigation. In Problem 2.1 students determine how long a race between two brothers should be if the younger (and slower) brother gets a head start. This introduces the y-intercept as a special point on a line, a pair of values in a table, or as the constant b in the equation y = ax + b. In Problem 2.2 they determine which cost plans for two different T-shirt companies that are represented by equations they should use for their walkathon. In so doing they use graphs and tables to find solutions to the equations and they connect the idea that an ordered pair of numbers for a point on the graph or table is a solution to an equation. In Problem 2.3 they look at pledge plans from sponsors that are represented by equations. They consider the advantages and disadvantages of using tables, graphs, or symbolic methods to solve equations of the form y = ax + b.
At the end of this Investigation, they find the rate of change, decide whether relationships are decreasing or increasing, and make connections among ordered pairs on a line, a pair of values in a row of a table, and the solution of a linear equation. They use tables, graphs, and numerical reasoning to solve linear equations and inequalities. They continue to explore which linear relationships are also proportional.
Investigation 3: Solving Equations Symbolically
Students continue to make the connection between points on a line, pairs of data points in a table, and solutions to equations. In Problem 3.1 linear equations are represented by pouches (the variable) and coins (the constant). They find the number of coins in a pouch by solving these pictorial equations by informally using Properties of Equality to write equivalent equations. In Problem 3.2 they represent pictorial situations of pouches and coins symbolically and encounter equivalent expressions for a given situation. They use the Distributive Property to show that the two expressions are equivalent. In Problem 3.3 they deepen their understanding of solving linear equations using equations devoid of context. They end the Problem by looking at a real-life application of linear equations from forensic science. In Problem 3.4 they look at two equations, one for the expenses to make cakes and the other for the income generated by selling the cakes. They explore how many cakes are needed to break even. They can do this graphically by finding the point of intersection of the two graphs or by using a table and finding when the two tables have the same entries. They also find the break-even point by setting the expression for expense equal to the expression for income and solving the equation for the number of cakes need to make the equation true. Note that by finding the point of intersection of two lines they are also solving a system of two linear equations, which is studied more formally in the 8th grade unit It’s in the System.
At the end of this unit, students use the properties of equality for solving equations in pictorial form and then transition into solving equations in symbolic form. They add or subtract the same number or variable or multiply or divide by the same nonzero number or variable on both sides of an equation. They also solve linear inequalities and use their solutions to answer questions about real‐world contexts. They continue to explore which linear relationships are also proportional.
Investigation 4: Connecting Rates and Ratios
Students continue to strengthen their understanding of rate and ratio by connecting the patterns of change between two variables that have a linear relationship to the ratio of the vertical distance to horizontal distance between two points on the graph of the relationship. In Problem 4.1 they find the ratio of vertical change to horizontal change between two points on a line by first exploring the steepness of a set of stairs. The connection between this ratio and rate of change is made explicit in Problem 4.2 as they explore the slope of a line as represented in a table, graph, and equation. They also explore how the rate of change of a linear relationship relates to the constant of proportionality. In Problem 4.3 they pull all the ideas of the unit together by looking at a new context that involves how many students are needed to attend a movie so that the theater’s income will equal its operating expense. They examine several student strategies for solving the problem.
At the end of this Investigation students find the slope of a line given two points on the line and then find the y‐intercept using either a table or a graph. They write an equation of the form y = ax + b, in which a is the slope and b is the y‐intercept. The unit ends with an application that involves students applying their knowledge of linearity. They use tables, graphs, and numerical reasoning to solve linear equations and inequalities. They continue to explore which linear relationships are also proportional.
Goals of the Units
Linear Relationships: Recognize problem situations in which two variables have a linear relationship
- Identify and describe the patterns of change between the independent and dependent variables for linear relationships (proportional and non-proportional) represented by tables, graphs, equations, or contextual settings.
- Recognize that when a linear relationship includes (0,0) it is a proportional relationship. Identify proportional relationships given a table, graph, equation, or real-world context.
- Identify the rate of change between two variables and the x- and y-intercepts from graphs, tables, and equations that represent linear relationships, and in proportional relationships, y = ax, recognize that a is the constant of proportionality or unit rate.
- Construct tables, graphs, and equations that represent linear relationships (proportional and non-proportional) given specific pieces of information from real world and mathematical contexts. Describe what information the variables and numbers represent.
- Translate information about linear relationships given in a contextual setting, a table, a graph, or an equation to the other forms.
- Make a connection between slope as a ratio of vertical distance to horizontal distance between two points on a line and the rate of change between two variables that have a linear relationship.
- Solve problems and make decisions about linear relationships using information given in tables, graphs, equations, and contextual situations.
Algebraic Expressions and Equations: Understand the relationship between expressions and equations
- Use properties of operations, including the Distributive Property to generate linear equivalent expressions and equations.
- Recognize that two linear equations are equivalent if they have identical solutions.
- Recognize that equivalent expressions can reveal different information about a situation and how the quantities are related.
- Recognize that the equation y = ax + b represents a linear relationship and that ax + b is an expression equivalent to the expression y.
- Recognize that linear equations in one unknown, k = ax + b or y = a(t) + b, where k, t, a, and b are constant numbers, are special cases of the equation y = ax + b.
- Recognize that finding the missing value of one of the variables in a linear relationship, y = ax + b, is the same as finding a missing coordinate of a point (x, y) that lies on the graph or table of the relationship.
- Recognize that a linear inequality in one unknown is associated with a linear equation.
- Solve linear equations and inequalities in one variable using symbolic methods, tables, and graphs.
Unit Alignments: Goals, CCSM, Arc Of Learning, Emerging Mathematics
Investigation 1: Walking Rates
Students will work to develop elements of the following Unit Goals throughout Investigation 1.
Linear Relationships: Recognize problem situations in which two variables have a linear relationship
- Identify and describe the patterns of change between the independent and dependent variables for linear relationships (proportional and non-proportional) represented by tables, graphs, equations, or contextual settings
- Recognize that when a linear relationship includes (0,0) it is a proportional relationship. Identify proportional relationships given the table, graph, equation, or real-world context
- Identify the rate of change between two variables and the x- and y-intercepts from graphs, tables, and equations that represent linear relationships, and in proportional relationships, y = mx, recognize that m is the constant of proportionality or unit rate
- Construct tables, graphs, and equations that represent linear relationships (proportional and non-proportional) given specific pieces of information from real world and mathematical contexts. Describe what information the variables and numbers represent
Algebraic Expressions and Equations: Understand the relationship between expressions and equations
- Recognize that the equation y = ax + b represents a linear relationship and means that ax + b is an expression equivalent to the expression y
- Recognize that finding the missing value of one of the variables in a linear relationship, y = ax + b, is the same as finding a missing coordinate of a point (x, y) that lies on the graph or table of the relationship
1.1 Walking Marathons: Using Rates
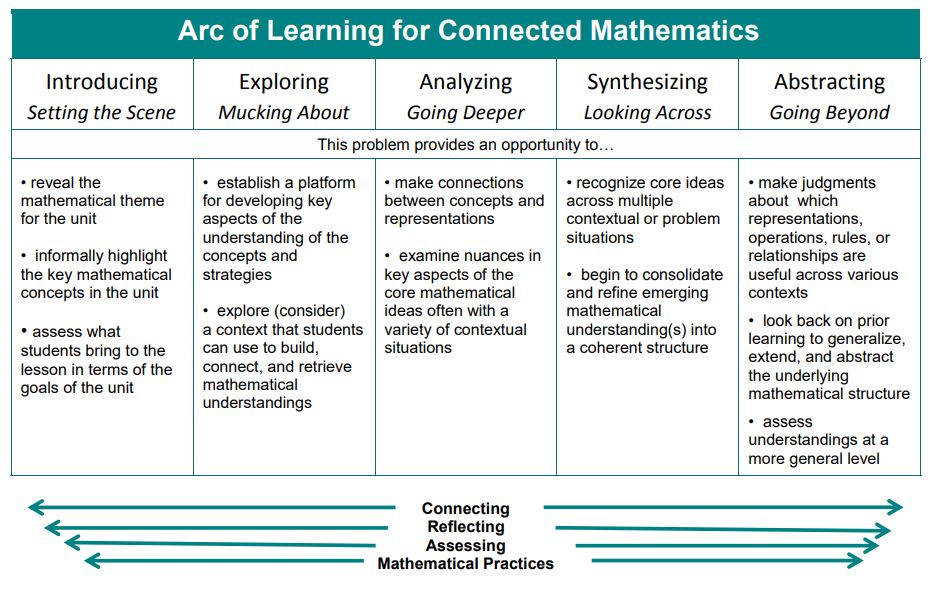
Arc of Learning
Introduce & Introduce/Explore
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.EE.B.4
NWDYK
Assume you continue to walk at the rate determined in the Initial Challenge. Describe in words the distance you could walk in a given number of seconds. Then write an equation to represent this information.
Emerging Mathematical Ideas
Begin making sense of a problem context that involves a proportional relationship between two variables (time, distance) described initially in words and connecting it to an equation and a graph of the relationship.
Begin to notice/recognize:
- rate is a way to describe how far you can travel in a fixed interval of time – e.g., in two seconds I can move 20 feet, and use phrases like “consistent rate” and “constant rate” to describe relationships like this one,
- proportional relationships,
- an understanding of rates can be connected to prior experiences with ratios and proportional reasoning (multiplicative reasoning),
- the relationship between the length of time traveled and the distance covered at a particular rate can be described in words, e.g., if I walk at a rate of 5 meters per second, then I can find the distance I travel by multiplying the number of seconds I travel by 5 m/sec. (the unit rate),
- the distance traveled (d) at a constant rate (r) for a period of time (t) can be described by the equation d = r x t,
- the equation needed to describe the distance traveled for two different people will be the same except that the value for the rate will vary, and
- when points for several different values of time and distance traveled (at a given rate) are graphed, the points will form a straight line
1.2 Walking Rates and Linear Relationships: Tables, Graphs and Equations
Arc of Learning
Introduce & Explore
CCSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d, 7.EE.B.4
NWDYK
How do you recognize a linear pattern of change in a verbal context, table, a graph, expression, or an equation?
Emerging Mathematical Ideas
Begin to make sense of linear patterns of change such as distance, and time (for given rates) including how to identify them and how to represent them in words, in a table, in a graph, as equivalent expressions, and as an equation connecting to prior experiences with proportional relationships.
Begin to notice:
- the connection between equivalent expressions and an equation, that is, an equation equates two or more equivalent expressions,
- how a constant rate of change is represented in a verbal context, table, graph, and equation,
- the ways different representations of linear patterns (tables, graphs, or equations) are more or less useful in easily answering questions depending on the nature of the question (e.g., you can quickly see the rate of change from an equation, but the graph of a relationship is a quick way to see if the relationship is linear),
- the characteristics in a table, graph and equation that can be used to identify a proportional relationship and the constant of proportionality (unit rate), and
- how variations in the way axes of a coordinate graph are partitioned can make the graph of a relationship look very different e.g., steeper or flatter lines.
1.3 Raising Money: Using Linear Relationships to Solve Equations
Arc of Learning
Explore & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d, 7.EE.B.4
NWDYK
How can you determine if a relationship is linear from a context, table, graph, or equation?
If a relationship is linear, explain how you can tell if it is proportional?
Emerging Mathematical Ideas
Expand understanding of linear and proportional relationships exploring a new context (miles walked, dollars raised) while using multiple representations to answer a variety of relevant questions.
Explore the Walkathon Fundraising context working to:
- identify independent and dependent variables in a real-world situation,
- represent linear relationships in tables, graphs, and equations give descriptions in words,
- make sense of an initial value in a linear relationship (a fixed donation) compared to a constant rate of change (dollars for each mile walked) and how each are represented in tables, graphs, equations and in the words describing the relationship,
- distinguish between linear and proportional relationships described in words, tables, graphs and equations (e.g., proportional relationships have a constant rate of change and must contain the point (0,0), where-as linear relationships have a constant rate of change, but may or may not contain the point (0,0)),
- interpret meaning of data points on graphs, in tables, and satisfying equations, and
- use and compare multiple linear relationships to answer relevant questions.
1.4 Walkathon Money: Expressing Linear Relationships and Proportionality
Arc of Learning
Explore & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d, 7.EE.B.4
NWDYK
Compare the patterns of change for the linear relationships in this Problem to those in previous Problems in this Investigation. Do any of these represent proportional situations? Explain.
Emerging Mathematical Ideas
Continue to explore and analyze linear relationships using a new decreasing context (week #, dollars withdrawn from account) that is initially presented with tabular, graphic, and symbolic representations.
Make sense of two decreasing linear relationships initially presented in tabular and graphic forms working to:
- recognize proportional and non-proportional linear relationships presented in tabular and graphic forms,
- identify the decreasing rates of change from the table and graph,
- identify the initial value of the relationship from the graph (y-intercept), and the table (y value when x = 0),
- describe these relationships in words and with equations and inequalities using one or more equivalent forms, and
- use the various representations to compare relationships and to efficiently answer relevant questions for each relationship.
Mathematical reflection
Arc of Learning
Explore & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d, 7.EE.A.2, 7.EE.B.4
NWDYK
What do you know about linear expressions, equations, inequalities, and relationships?
Emerging Mathematical Ideas
Work to build and connect mathematical understandings for key aspects of linear relationships that are presented in a variety of representations.
Notice that:
- a constant rate of change is a key characteristic of linear relationships (both proportional and non-proportional),
- the constant rate of change in a linear relationship can be identified from the table, graph, equation or text,
- the constant rate of change in a linear relationship (both proportional and non-proportional) can be positive, negative, or zero,
- the initial value of a linear relationship can be identified from the graph (y-intercept), the table (y value when x = 0), and the equation (value of the constant term b in y = mx + b or y = mx),
- when the initial value (b = 0) of a linear relationship is 0, the relationship is proportional,
- linear relationships (both proportional and non-proportional) can be represented with words, equations, table and graphs,
- the various representations of linear relationships can be used to compare relationships and to efficiently answer relevant questions for each relationship.
Note: A constant rate of change means that as the independent variable increases by a constant amount (x = 1, 2, 3, 4, ….), the corresponding dependent variable will change by a constant amount.
Investigation 2: Using Tables, Graphs, and Equations to Explore Linear Relationships
Students will be working to develop elements of the following Unit Goals throughout Investigation 2.
Linear Relationships: Recognize problem situations in which two variables have a linear relationship
- Identify and describe the patterns of change between the independent and dependent variables for linear relationships (proportional and non-proportional) represented by tables, graphs, equations, or contextual settings
- Recognize that when a linear relationship includes (0,0) it is a proportional relationship. Identify proportional relationships given the table, graph, equation, or real-world context
- Identify the rate of change between two variables and the x- and y-intercepts from graphs, tables, and equations that represent linear relationships, and in proportional relationships, y = mx, recognize that m is the constant of proportionality or unit rate
- Construct tables, graphs, and equations that represent linear relationships (proportional and non-proportional) given specific pieces of information from real-world and mathematical contexts. Describe what information the variables and numbers represent
- Translate information about linear relationships given in a contextual setting, table, graph, or equation to the other forms
- Solve information about linear relationships given in a contextual setting, table, graph, or equation to the other forms
Algebraic Expressions and Equations: Understand the relationship between expressions and equations
- Recognize that the equation y = ax + b represents a linear relationship and means that ax + b is an expression equivalent to the expression y
- Recognize that finding the missing value of one of the variables in a linear relationship y = ax + b is the same as finding a missing coordinate of a point (x, y) that lies on the graph or table of the relationship
2.1 Henri and Emile's Race: Developing Strategies to Find a Solution
Arc of Learning
Explore/Analysis & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.RP.A.2c, 7.EE.A.1, 7.EE.A.2, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
NWDYK
How can a table, graph, and equation that represents a linear relationship be used to answer questions about the linear relationship? What other strategies could you use?
Emerging Mathematical Ideas
Make sense of a new problem context with two contrasting linear relationships and examine the use of tables, graphs, equations, and inequalities to find solutions.
Look for patterns in various representations that can be used to answer questions about rate of change for linear relationships such as:
- in tables, the rate of change is the difference between y values when the table values for x change at a constant rate of one,
- the direction of the slant of the line in a graph lets you know if the rate of change is positive (lines where the y values are increasing) or negative (where the y values are decreasing), and
- the steepness of the line (going up or down) lets you know about the magnitude of the rate of change (e.g., a very steep line implies a large, positive or negative rate of change; where the line is barely increasing or decreasing, you would expect to see smaller values for the rate of change),
- in the equation of a line the rate of change is the number that multiplies the independent variable (for example in Problem 2.1 it is the number of meters traveled each second with time as the independent variable), and
- identify and represent proportional relationships as tables, graphs, and equations and distinguish from non-proportional linear relationships.
Look for patterns in various representations that can be used to find the value of one variable in a linear relationship when you have a value for the other variable such as:
- in a table, corresponding values for independent and dependent variable are side-by-side or above and below depending on the orientation of the table,
- in a graph, the coordinates of points on the line provide the values of corresponding independent and dependent variables, and
- in an equation or inequality, the value of the dependent variable can be found for any value of the independent variable by performing the indicated operations in the equation or inequality.
Notice that informal guess and check strategies may allow students to answer questions about when two related linear relationships have the same value such as how much time has passed (t) for the distances traveled (d) to be the same for both brothers.
NOTE: In an equation, it is not yet true that students will be able to find the value of the independent variable given a value for the dependent variable (e.g., solve 100 = 2x + 45).
2.2 Comparing Cost Relationships: Using Tables and Graphs to Find Solutions to Equations
Arc of Learning
Analysis & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.EE.A.1, 7.EE.A.2, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
NWDYK
How do you know if the ordered pair of numbers for a point on a graph is a solution to an equation? How can you use a table or graph to find a solution for an equation?
Emerging Mathematical Ideas
Continue examining ways tables, graphs, equations, and inequalities can be used to find solutions, using a new problem context presented in the form of two contrasting linear equations.
Look for patterns that can be used to find solutions to linear relationships including:
- using a completed table, look for the value of the dependent variable (y) that corresponds to the needed value of the independent variable (x),
- using a table that is incomplete, substitute the value for the independent value (n) into the given equation and perform the indicated operations to find the corresponding value for the dependent variable (CM) (solution) (e.g., for CM = 49 + n, to find n = 100, the solution, CM, is equal to 49 + 100 = 149),
- in a graph, the coordinates of the points (a,b) on the line represent all of the solutions to the equation of the line; to find the solution for a given value of the independent variable (first coordinate a), find the point on the line with the first coordinate and read the corresponding b value in the ordered pair, and
- in an equation or inequality, the value of the dependent variable (y) can be found for any value of the independent variable (x) by substituting the needed value of x into the equation and performing the indicated operations in the equation to find the solution (y).
Identify and represent proportional relationships as tables, graphs, and equations and distinguish from non-proportional linear relationships.
2.3 Connecting Tables, Graphs, and Equations to Solutions
Arc of Learning
Analysis & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.EE.A.1, 7.EE.A.2, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
NWDYK
Describe the advantages and disadvantages of using tables, graphs, and symbolic methods to solve equations
Emerging Mathematical Ideas
Continue working to develop strategies for finding solutions for linear relationships using multiple representations including: graphs, tables, equations, and inequalities. The focus of Problem 2.3 is to make connections between solutions found using one representation (e.g., graphs) to each of the other representations (e.g., tables and equations) where they are also visible.
Look for patterns connecting solutions for a linear relationship found on a graph, table, equation, or inequality to solutions found using other representations including:
- the coordinates of a point (a,b) on the graph of a line is a solution for the linear relationship. The ordered pair (a,b) can also be found as a corresponding pair of values on a table representing solutions for the line, and when the equation is evaluated at x = a, the value found for y will be equal to b.
Notice that answering some questions about a linear relationship can be easier or harder depending on the representation. For example,
- it can be harder to find rate of change for a linear relationship on a graph or table than it is to find it using an equation, or
- it can be easier to find the solution (y value) for a linear relationship for a given value of the independent variable using a table or a graph.
Identify and represent proportional relationships as tables, graphs, and equations and distinguish from non-proportional linear relationships
Mathematical Reflection
Arc of Learning
Analysis & Analysis
CCSSM
7.RP.A.2, 7.RP.A.2a, 7.EE.A.1, 7.EE.A.2, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
Mathematical Reflection
What do you know about linear expressions, equations, inequalities, and relationships?
Emerging Mathematical Ideas
Linear relationships (proportional and non-proportional) found in real-world situations can be represented using equations, inequalities, tables, or graphs. Connections can be made between each of these representations and the rate of change of the linear relationship.
Each representation of a linear relationship (proportional and non-proportional) can be used to find the rate of change for the relationship including:
- using an equation, y = ax + b or y = ax, the rate of change (constant of proportionality) is the multiplier of the independent variable (a),
- in tables the rate of change (constant of proportionality) is the difference between y values (dependent variable) when the table values for x (independent variable) change at the constant rate of one,
- on a graph the direction of the slant of the line lets you know if the rate of change is positive (lines where the y values are increasing as x increases) or negative (where the y values are decreasing as x increases), and
- the steepness of the line (going up or down) lets you know about the magnitude of the rate of change (e.g., a very steep line implies a large, positive or negative rate of change; where the line is barely increasing or decreasing, you would expect to see smaller values for the rate of change).
Each representation of linear relationships can be used to identify proportional versus non-proportional linear relationships including:
- the graph of a line that passes through the point (0,0) represents a proportional relationship,
- the graph of a line with a y-intercept that is not (0,0) represents a non-proportional relationship,
- tables that include 0,0 as an ordered pair and that have a constant rate of change in the dependent values
- when the table values for x (independent variable) change at the constant rate of one, and
- equations of the form y = ax represent proportional relationships.
Each representation of linear relationships (proportional and non-proportional) can be used to find solutions for the relationship including:
- a solution (a,b) that satisfies the equation of a line y = x + m or y = mx can also be found as an ordered pair in the table of values representing the linear relationship, and as a point on the line that is a graph of the relationship,
- a corresponding pair of values (a,b) found on a table representing solutions for a linear relationship can likewise be found as a point on the graph of the line, and when the equation is evaluated at x = a, the value found for y will be b, and
the coordinates of a point (a,b) on the graph of a line is a solution for the linear relationship. The ordered pair (a,b) can also be found as a corresponding pair of values on a table representing solutions for the line and when the equation is evaluated at x = a, the value found for y will be equal to b.
Investigation 3: Solving Linear Equations Symbolically
Students will be working to develop elements of the following Unit Goals throughout Investigation 3.
Linear Relationships: Recognize problem situations in which two variables have a linear relationship
- Recognize that when a linear relationship includes (0,0) it is a proportional relationship. Identify proportional relationships given the table, graph, equation, or real-world context
- Identify the rate of change between two variables and the x- and y-intercepts from graphs, tables, and equations that represent linear relationships, and in proportional relationships y = mx, recognize that m is the constant of proportionality or unit rate
- Construct tables, graphs, and equations that represent linear relationships (proportional and non-proportional) given specific pieces of information from real-world and mathematical contexts. Describe what information the variables and numbers represent
- Translate information about linear relationships given in a contextual setting, table, graph, or equation to the other forms
- Solve problems and make decisions about linear relationships using information given in tables, graphs, equations, and contextual situations
Algebraic Expressions and Equations: Understand the relationship between expressions and equations
- Use properties of operations and the Distributive Property to generate linear equivalent expressions and equations
- Recognize that two linear equations are equivalent if they can be represented by identical tables or graphs
- Recognize that equivalent expressions can reveal different information about a situation and how the quantities are related
- Recognize that the equation y = ax + b represents a linear relationship and means that ax + b is an expression equivalent to the expression y
- Recognize that linear equations in one unknown, k = ax + b or y = a(t) + b, where k, t, a, and b are constant numbers, are special cases of the equation y = ax + b
- Recognize that finding the missing value of one of the variables in a linear relationship y = ax + b is the same as finding a missing coordinate of a point (x, y) that lies on the graph or table of the relationship
- Recognize that a linear inequality in one unknown is associated with a linear equation
- Solve linear equations and inequalities in one variable using symbolic methods, tables, and graphs
3.1 Mystery Pouches: Exploring Equality
Arc of Learning
Explore & Explore
CCSSM
7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.4, 7.EE.B.4a
NWDYK
Pouches that contain an unknown number of coins as well as individual coins are represented with an equation. What strategies can you use to find the number of coins in each pouch? How do you know if your answer is correct?
Emerging Mathematical Ideas
Begin to make sense of algebraic equations presented as coins (numbers) and pouches (variables) and explore approaches to solving them.
Begin exploring algebraic strategies to solve equations modeled with pouches and coins by:
- making sense of equations modeled with pouches and coins as variables and numbers,
- translating between algebraic representations of equations and models,
- noticing that there may be multiple equivalent forms for an expression or equation, and
- using prior experiences with the Distributive Property, fact families, and numeric reasoning to make sense of steps needed to solve equations represented by models.
3.2 From Pouches to Variables: Writing and Solving Equations
Arc of Learning
Analysis & Explore
CCSSM
7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.4, 7.EE.B.4a
NWDYK
Describe a strategy for solving equations using what you know about equality. How do you know if your answer is correct?
Emerging Mathematical Ideas
Explore algebraic approaches to solving equations based on prior experiences with pouches and coins using the Distributive Property, fact families, and properties of operations and equality.
Build on experiences with pouches and coins to explore algebraic strategies for solving equations including:
- noticing that the Distributive Property allows you to create multiple equivalent forms for an expression or equation,
- applying the Distributive Property and fact families (composing/decomposing numbers) to justify steps needed to solve equations (find the number of coins in each pouch), and
- applying the properties of operations and equality (subtract or divide both sides of an equation by the same number) and fact families to justify steps needed to solve equations.
Notice that you can check to see if you have found the correct number of coins in a pouch (variable) by replacing each pouch (variable) in the original clue/equation with your answer and simplifying to see if the two sides are equal.
3.3 Solving Linear Equations
Arc of Learning
Analysis & Explore/Analysis
CCSSM
7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
NWDYK
Describe the strategies you can use to solve linear equations using the properties of operations and equality.
Emerging Mathematical Ideas
Extend and apply strategies for solving equations algebraically to equations with integer, fractional, and decimal values (coefficients and constants).
Find the solutions to linear equations using symbolic methods (properties of operations and equality) including:
- identifying and applying needed operations to both sides (such as adding 10 to both sides or dividing both sides by 2.5) in order to keep the expressions on each side of the equation equal,
- identifying when to apply the Distributive Property as needed to reach a solution efficiently (factored or expanded),
- applying order of operations appropriately as needed to simplify equations, and
- understanding the goal is to isolate the variable on one side of the equation.
Connect newly developed symbolic methods for solving equations (answering questions about relationships) to prior experiences with solution strategies that use tables and graphs including:
- recognizing that equations with linear expressions on both sides of the equal sign (2x – 5 = x + 7) can be viewed as two equations (y = 2x - 5 and y = x + 7), where the solution to the original equation (2x – 5 = x + 7) is the point where the two lines intersect on the graph,
- strategically selecting an efficient method to use for a given situation such as when a symbolic approach would be quick or when a calculator could be easily used to generate a graph or table to answer many questions about the relationship, and
- generating questions for a given relationship and making sense of questions asked about variables for a given equation.
3.4 The Point of Intersection: Using Equations and Inequalities
Arc of Learning
Analysis & Analysis
CCSSM
7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
NWDYK
Describe how you can solve an equation of the form ax + b = y?
Describe how you can solve an inequality of the form ax + b < c or ax + b > c?
Emerging Mathematical Ideas
Extend and apply strategies for solving equations using symbols and graphs to solve two related equations and to answer questions about the relationships.
Use symbolic and graphical strategies to answer questions about two linear relationships with the same independent variable including:
- using symbolic methods to find the value for x where the two equations are equal (that is have the same y value),
- understanding the meaning of the point where the graphs of the two equations intersect for a given context, and
- using graphs and equations to answer questions about the two related equations such as when one equation is greater or less than the other.
Mathematical Reflections
Arc of Learning
Analysis & Analysis
CCSSM
7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
Mathematical Reflection
What do you know about linear expressions, equations, inequalities, and relationships?
Emerging Mathematical Ideas
Linear relationships (proportional and non-proportional) in real-world and mathematical situations with positive and negative rational numbers can be represented using equations, inequalities, tables, or graphs. Each representation can be used to answer questions about the relationship. The focus of this Investigation was solving linear equations and inequalities symbolically using the Distributive Property, properties of operations, and numeric reasoning.
Analyze and use multiple representations to answer questions about a linear relationship (proportional and non-proportional) including:
- finding the value of one variable when given a value for the other variable represented as an equation, graph, or table (e.g., using an equation if y = 3x + 4 and y = 10, then to find the corresponding value for x you substitute 10 in place of y and simplify to find x = 2),
- answering questions about inequalities represented as a graph, table, or symbolically (e.g., find the values that satisfy an inequality such as 3x + 4 < 10, graph the corresponding linear relationship (y = 3x + 4) and then find the points on the line whose y-values are less than 10), and
- answering questions about two related linear relationships such as when one is greater/less than the other, or when they have the values for x and y, for relationships represented as graphs or equations.
Use the Distributive Property, properties of operations, and numeric reasoning to create multiple equivalent forms for an expression or equation as needed to simplify or reason about the relationship.
Investigation 4: Connecting Rates and Ratios
Students will be working to develop elements of the following Unit Goals throughout Investigation 4.
Linear Relationships:Recognize problem situations in which two variables have a linear relationship
- Identify and describe the patterns of change between the independent and dependent variables for linear relationships (proportional and non-proportional) represented by tables, graphs, equations, or contextual settings
- Recognize that when a linear relationship includes (0,0) it is a proportional relationship. Identify proportional relationships given the table, graph, equation, or real-world context
- Identify the rate of change between two variables and the x- and y-intercepts from graphs, tables, and equations that represent linear relationships, and in proportional relationships y = mx, recognize that m is the constant of proportionality or unit rate
- Construct tables, graphs, and equations that represent linear relationships (proportional and non-proportional) given specific pieces of information from real-world and mathematical contexts. Describe what information the variables and numbers represent
- Translate information about linear relationships given in a contextual setting, table, graph, or equation to the other forms
- Solve problems and make decisions about linear relationships using information given in tables, graphs, equations, and contextual situations
Algebraic Expressions and Equations: Understand the relationship between expressions and equations
- Use properties of operations and the Distributive Property to generate linear equivalent expressions and equations
- Recognize that two linear equations are equivalent if they can be represented by identical tables or graphRecognize that equivalent expressions can reveal different information about a situation and how the quantities are related
- Recognize that the equation y = ax + b represents a linear relationship and means that ax + b is an expression equivalent to the expression y
- Recognize that linear equations in one unknown k = ax + b or y = a(t) + b, where k, t, a, and b are constant numbers, are special cases of the equation y = ax + b
- Recognize that finding the missing value of one of the variables in a linear relationship y = ax + b, is the same as finding a missing coordinate of a point (x, y) that lies on the graph or table of the relationship
- Recognize that a linear inequality in one unknown is associated with a linear equation
- Solve linear equations and inequalities in one variable using symbolic methods, tables, and graphs
4.1 Climbing Stairs: Using the Ratio of Rise to Run
Arc of Learning
Analysis & Analysis
CCSSM
7.RP.A, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c
NWDYK
How does the steepness of stairs expressed as a ratio relate to a straight-line graph? To a linear equation that represents the graph?
Emerging Mathematical Ideas
Begin to analyze linear relationships (proportional and non-proportional) to understand how the constant rate of change can be understood and represented as a ratio.
Notice that the:
- ratio of the rise (height of each step) to the run (the depth of each step) is the same for each step along a staircase,
- the steeper the staircase the bigger the ratio of rise to run for each step and vise-versa, and
- the constant rate of change in every linear relationship (represented as graphs or equations) can be described as the ratio of the vertical change to the horizontal change for any section of the graph.
4.2 A Constant of Proportionality
Arc of Learning
Analysis/Synthesis & Analysis
CCSSM
7.RP.A, 7.RP.A.1, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d
NWDYK
How is the rate of change of a linear relationship related to the constant of proportionality?
Emerging Mathematical Ideas
Make connections, where appropriate, among the rate of change for linear relationships, the constant of proportionality for proportions, and the constant ratio of the vertical change to horizontal change for linear relationships using a variety of representations.
Notice that:
- some linear relationships are proportional and others are not,
- the rate of change describes how one variable in the relationship changes with respect to the other variable and can be found using a table of values, graph, or equation,
- for linear relationships that are also proportional, the rate of change, the constant of proportionality, the ratio of vertical change to horizontal change, and the unit rate of change are all the same, and
- the ordered pair (1, a) for a proportional relationship represents the unit rate of change for the relationship (that is a/1) where a is the constant of proportionality, and the equation of the line is y = ax.
4.3 Putting It All Together
Arc of Learning
Synthesis & Synthesis
CCSSM
7.RP.A, 7.RP.A.1, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d
NWDYK
How do the situations in this problem compare to other problems in this unit? Do any of these represent proportional situations? Why?
In what ways are the tables, graphs, equations, and inequalities useful for solving problems?
Emerging Mathematical Ideas
Work to consolidate and refine emerging key mathematical concepts associated with linear relationships, both proportional and non-proportional, into a coherent constellation of ideas.
Recognize that:
- linear relationships are identified by their constant rate of change that can be found using a problem context, table of values, graph, or equation,
- some linear relationships are proportional, and others are not,
- proportional relationships will always include the value (0,0), and will be of the form y = ax where a is the constant of proportionality, and
- when given the value of one variable for a linear relationship you can find the value of the other variable strategically using a variety of representations including graphs, tables, and equations.
Mathematical Reflection
Arc of Learning
Synthesis & Synthesis
CCSSM
7.RP.A, 7.RP.A.1, 7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b, 7.RP.A.2c, 7.RP.A.2d, 7.NS.A.2c, 7.EE.A, 7.EE.A.1, 7.EE.A.2, 7.EE.B, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a, 7.EE.B.4b
Mathematical Reflection
What do you know about linear expressions, equations, inequalities, and relationships?
Emerging Mathematical Ideas
Consolidate and refine key mathematical concepts associated with linear relationships, both proportional and non-proportional, into a coherent constellation of concepts and skills.
Recognize that:
- rate of change describes how one variable in the relationship changes with respect to the other variable,
- linear relationships have a constant rate of change that can be identified using the problem context, table of values, graph, or equation,
- the rate of change describes the steepness of a line and the ratio of the vertical change to the horizontal change for any section of the graph,
- some linear relationships are proportional, and others are not and be able to distinguish between the two,
- proportional relationships will always include the value (0,0), and will be of the form y = ax where a is the constant of proportionality,
- when given the value of one variable for a linear relationship you can find the value of the other variable using a variety of representations including graphs, tables, and equations, and
- the Distributive Property, properties of operations, and numeric reasoning can be used to create multiple equivalent forms for an expression or equation as needed to simplify or reason about the relationship.
Assessments
Check-Up #1
#1 a. 7.RP.A.1, 7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b
b. 7.RP.A.2
c. 7.RP.A.2c, 7.RP.A.2d
d. 7.RP.A.2c, 7.RP.A.2d
#2 a. 7.RP.A.1, 7.RP.A.2, 7.RP.A.2a, 7.RP.A.2b
b. 7.RP.A.2, 7.RP.A.2c
#3 7.RP.A.2, 7.RP.A.2a
Partner Quiz
#1 a. 7.RP.A.2, 7.RP.A.2b, 7.RP.A.2a
b. 7.RP.A, 7.RP.A.2, 7.EE.B
c. 7.RP.A, 7.RP.A.2, 7.EE.B
#2 7.RP.A, 7.RP.A.2, 7.RP.A.2c, 7.RP.A.3, 7.EE.B.4, 7.EE.B.4a
#3 a. 7.RP.A.2
b. 7.RP.A.2b
c. 7.RP.A.2c
d. 7.RP.A.2c
e. 7.RP.A.3.2d
Check-Up #2
#1 a. 7.EE.B, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a
b. 7.EE.B, 7.EE.B.3, 7.EE.B.4, 7.EE.B.4a
c. 7.RP.A.2, 7.RP.A.2a
#2 a. 7.EE.B, 7.EE.B.3
#3 7.EE.B.3
#4 7.EE.B.3
Unit Test
#1 a. 7.RP.A.2b
b. 7.RP.A.2c
c.7.RP.A, 7.RP.A.2a
#2 a. 7.RP.A.2, 7.RP.A.2a
b. 7.RP.A.2, 7.RP.A.2b
#3 a. 7.RP.A.2, 7.RP.A.2b
b. 7.RP.A.2 , 7.RP.A.2b, 7.RP.A.2d
c. 7.RP.A.2, 7.RP.A.2a
d. 7.RP.A, 7.EE.B.4a or b
f. 7.RP.A.2d
#4. Regardless of the representation students choose to use to solve #4 a-c, they may use: 7.RP.A.2c, 7.RP.A.2d, 7.RP.A.3
Depending on the representation students choose to use to solve #4 a-c, they may use: 7.RP.A.2c, 7.RP.A.2d, 7.EE.B4 and 7.EE.B.4a
#5 a. 7.EE.B.3
b. 7.EE.B.3
#6 7.EE.B.3
General Arc of Learning
Unit Arc of Learning
This unit continues the exploration of analyzing and representing quantitative relationships between independent and dependent variables that was started in the 6th grade Variables and Patterns unit by using linear relationships as the context. Using linear situations as a context naturally allows an exploration of what it means to be linear and provides an opportunity to strengthen students’ understanding and use of linear expressions and linear equations. It also strengthens students’ understanding of proportional relationships as they look at linear situations, some of which are proportional relationships, and some are not. Linear expressions and equations will be used to study and represent general properties of geometry, data, and probability in the 7th grade units: Filling and Wrapping; What Do You Expect?; and Samples and Populations. Proportional relationships also continue in these 7th grade units. More work with linear relationships, expressions, and equations is done in several Units in 8th grade: Thinking with Mathematical Models; Growing, Growing, Growing; Frogs, Fleas and Painted Cubes; Say It with Symbols; and It’s in the System.
Mathematical Overview
Mathematics Overview: Moving Straight Ahead
Key ideas: Linearity, representations of linear relationships, rate of change, slope, meaning of equation, solving equations, equivalent expressions and equations, solving inequalities
Major Focus: Algebraic Reasoning
Strategic Connections: Proportional Reasoning
Moving Straight Ahead follows up the groundwork laid in Variables and Patterns. In that unit students learned to represent relationships between two variables, using tables, graphs, and equations, and to use these representations to solve problems. Subsequent 6th and 7th grade units provided varying contexts in which to practice these ideas. Moving Straight Ahead bores down on the idea of linearity. The unit focuses on the unique characteristics of linear relationships and how to recognize these in tables, graphs, and equations. In particular, the constant rate of change of the dependent variable as the independent variable changes provides connections among the representations. Slope is defined as a ratio of geometric measures and connected to rate of change. Students solve one-variable linear equations using tables, graphs, and properties of equality. Equivalent expressions arise as students write equations to represent relationships, and as they solve linear equations. Inequalities are introduced and solution strategies are developed.
Link to the Future: In 8th grade students will continue to study linear relationships, and compare them to non-linear relationships, such as inverse proportions and quadratics. The symbolic work with expressions and equations will continue to be expanded with linear and non-linear equations and expressions.
In roughly this order, students:
- Define what it means for a relationship to be linear.
- Recognize linearity in the representations of a relationship.
- Move between representations.
- Reinforce the understanding that some linear relationships are proportional.
- Make sense of the components of a linear equation.
- Connect rate of change in a context, table, graph, and equation.
- Connect solutions to linear equations to points on a graph or ordered pairs in a table.
- Solve problems involving linear equations using symbolic methods, tables, and graphs.*
- Understand and generate equivalent expressions.
- Solve linear inequalities by associating them with linear equations, and using tables, graphs, or symbolic reasoning.
- Connect rate of change to slope ratio.
*Note: Using properties of equality is the traditional way to solve linear equations. As students progress in their experience with algebra, they will encounter non-linear equations, some of which cannot be solved symbolically. Graphs and tables can always be used to solve for real solutions. For example: 3x + x = 100 can be graphed and solved, but cannot solved symbolically.
Linearity: Identifying Linear Relationships and Connecting Representations
How Linear Relationships are Recognized
Constant Rate of Change
In a linear relationship, as the value of the independent variable changes by a set amount the value of the dependent variable changes by a constant amount. This results in a straight-line graph. Variables in a non-linear relationship do not show a constant rate of change. The constant rate of change can be recognized in the table, graph, or equation.
For example, suppose we compare prices from two T-shirt printing companies. Mighty Tee charges $49 plus $1 per T-shirt. No Shrink charges $4.50 per T-shirt.
From the table we see the constant rate of change. This ratio or rate is the same no matter between which two ordered pairs we choose to measure it. This indicates a linear relationship.
.png)
For Mighty Tee the rate of change is 1/1 (or 2/2 or 8/8, etc.). In context this means that for every 1 more T-shirt ordered the cost increases by $1. For No Shrink the rate of change is 4.5/1 (or 9/2 or 31.5/7, etc.).
From the graph we see that the constant rate of change measures the steepness of the line. The ratio of (change in C) : (change in N) is the slope. The slope is the same no matter between which two points we choose to measure it.
.png)
The “steps” on the Mighty Tee graph show a ratio or slope 1/1 (or 6/6 or 11/11).
The “steps” on the No Shrink graph show a ratio or slope of 4.5/1 (or 31.5/7).
Note the scales on the axes are not the same.
Components of a Linear Equation/Relationship
There are two components of a linear relationship: the constant rate of change and the y-intercept. The y-intercept is the point at which the line crosses the y-axis (0, y). Both are visible in the table and graph and equation.
In the table on the left, we can see the constant rate of change for Mighty Tee is 1/1 or 1, and the y-intercept is (0, 49). The equation for relating cost and number of T’s for Mighty Tee is C = 1N + 49. All the pairs (N, C) in the table and on the associated graph are solutions for this equation, and all solutions for this equation are on the graph.
The constant rate of change for No Shrink is 4.5/1 or 4.5, and the y-intercept is (0,0). The equation relating cost and number is C = 4.5N + 0.
Linear relationships can always be written in the form y = ax + b, where a is the slope and (0, b) is the y-intercept.
Distinguishing Between Proportional and Non-Proportional Linear Relationships
For both T-shirt plans, Cost and Number are linearly related. However, only one is also a proportional relationship.
No Shrink
| Number | 0 | 1 | 2 | 3 | ... | 10 |
|---|---|---|---|---|---|---|
| Cost | 0 | 4.5 | 9 | 13.5 | ... | 45 |
We have already seen that the constant rate of change is 4.5/1. Notice also the equal vertical ratios in the table, $4.50:1, $9:2, $13.5:3, $45:10. That is, the vertical ratios C/N are the same as the constant rate of change. So, the rate of change is also the constant of proportionality.
Mighty Tee
| Number | 0 | 1 | 2 | ... | 10 | 11 |
|---|---|---|---|---|---|---|
| Cost | 49 | 50 | 51 | ... | 59 | 60 |
We have seen the constant rate of change is 1/1. Notice that the vertical ratios change, $50:1, $51:2, $59:10, $60:11 etc. The vertical ratios C/N are not the same as the constant rate of change. For this reason, there is no constant of proportionality.
Compare the Graphs
Notice that for the points on this line, (1, 4.5), (2, 9), (3, 13.5), (10, 45) etc., the ratio of coordinates C/N = 4.5/1. This is equivalent to the equation C = 4.5N. All proportional relationships have this characteristic that Y/X = constant rate of change. They can be written as Y/X = a or Y = aX, where a is the constant rate of change, constant of proportionality, or slope, and the y-intercept is (0,0).
For points on Mighty Tee’s graph, (1, 50), (2, 51), (11, 60) etc., the ratios of coordinates are not equal. The equation representing this relationship cannot be written C/N = a constant. It is not a proportional relationship. The y-intercept is not (0, 0).
Moving Between Representations
Starting with the equation and generating ordered pairs for the table or graph is just a matter of substituting values for one variable to find the related value for the other variable. It is also possible to start with a table or graph and find the related equation. See below for two examples.
.png) Negative Slope
Negative SlopeThe rate of change (or slope) is -2/1 (or -14/7). It is constant so this is a linear relationship. The intercept is (0, 16). The equation that represents this linear relationship is y = -2x + 16.
.png)
.png) Zero Slope
Zero SlopeThe rate of change (or slope) is 0/1 (or 0/7). It is constant, so this is a linear relationship. The intercept it (0, -3) so the equation is y = 0x – 3 (or just y = –3.)
.png) Negative Slope
Negative SlopeNote: A line may have a positive, negative, or zero slope, as illustrated above. A zero slope indicates a horizontal line. A line may also have an undefined slope. This occurs when the change in the independent variable is 0. For example, the points (4, 0), (4, 6), (4, 10) define a vertical line. We cannot measure its slope because the ratio would be (change in y)/(change in x) = (change in y)/0. Since we cannot divide by 0 and find a rational number, we say this slope is undefined.
Solutions for Linear Equations and Inequalities: Meaning, Strategies
Strategies for Solving Linear Equations
To solve a linear equation means to find values for the variable that make the equation true. Since the table and graph are representations of the equation, they are also representations of solutions for the equation. A solution of an equation is an ordered pair that makes the equation true and lies on the graph of the line.
Solving two-variable equations: Suppose we have an equation y = 3x + 49. Then, making a table we have:
| X | 0 | 1 | 2 | ... | 17 |
|---|---|---|---|---|---|
| Y | 49 | 52 | 55 | ... | 100 |
So, (0, 49), (1, 52), (2, 55), (17, 100) are solutions for y = 3x + 49. (These solutions would also appear as points on the graph of y = 3x + 49.)
Solving one-variable linear equations. Example: Solve 3x + 49 = 100.
3x + 49 = 100 is a specific case of the equation y = 3x + 49. 3x + 49 = 100 asks, “What is the value of x if the value of y is 100?”
Solving from the table: By substituting values in for x and making a table we will eventually come to the solution (as in the above table) that when x = 17, y = 100. So x = 17 satisfies 3x + 49 = 100.
Solving from the graph: By making a graph of y = 3x + 49 and looking for (x, 100) we find the solution for 100 = 3x + 49 at (17, 100).
Solving symbolically: This strategy involves rewriting the equation as a simpler, equivalent equation.
If 3x + 49 = 100, then the equal sign will still be true if we subtract 49 from both sides of the equation. That is, 3x = 51 will be just as
true, and have the same solutions, as the original equation.
Rebalancing the equation by dividing both sides by 3 we have x = 17.
This strategy takes advantage of the properties of equality: you can add, subtract, multiply or divide both sides of an equality and it will produce an equivalent equality.
Solving one-variable equations with x on both sides.
The equation for Mighty Tee is C = 1N + 49. The equation for No Shrink is C = 4.5N. If we want to know when the cost would be the same for both companies we have to solve 4.5N = 1N + 49.
Graphical solution:
.png)
The point (14, 63) lies on both graphs. It is a solution for C = 1N + 49 and C = 4.5N. That is, when N = 14, C = 63 for both equations or when the number of T-shirts is 14 the cost is $63 for both companies. When N = 14, 4.5N = 1N + 49.
Symbolic solution:
If 4.5N = 1N + 49 then subtracting 1N from each side, we have 3.5N = 49.
And dividing each side by 3.5, N = 14.
The Costs are equal when the Number of Ts is 14.
Strategies for Solving Linear Inequalities
Using a graph or a table
Example:
For the two T-shirt companies. We have C = 1N + 49 and C = 4.5N. 1N + 49 > 60 asks “What number of Ts would make the cost at Mighty Tee greater than $60?” We can solve this by examining a graph (or table) for points where the cost is greater than 60.
In the left column, we see (11, 60) on the graph of C = 49 + 1N. For values of N to the right of this point the cost is greater than $60, that is 49 + 1N > 60 for N > 11.
![]()
Example:
4.5N < 90 asks, “What number of Ts would make the cost at No Shrink less than $90?” Again, we can examine the table (or graph) to find points where the cost is less than $90.
| N | 0 | 1 | 2 | ... | 19 | 20 | 21 |
|---|---|---|---|---|---|---|---|
| C | 0 | 4.5 | 9 | ... | 85.5 | 90 | 94.5 |
When N < 20, C < 90.
![]()
Example:
1N + 49 < 4.5N asks, “For what number of Ts is the Cost at Mighty Tee less than the cost at No Shrink?” On the graph in the left column, we see that the Cost for Mighty Tee is lower than the cost for No Shrink when N >14.
![]()
Note: The number of Ts has to be a whole number, so we want the graph of the solution set to show only positive whole numbers.
Solving symbolically by using the equation:
It is also possible to solve 1N + 49 < 4.5N symbolically.
1N + 49 < 4.5N is related to 1N + 49 = 4.5N.
Solving the equation symbolically we saw that the solution for 1N + 49 = 4.5N is N = 14.
When N = 14 the costs are equal.
Now check costs near N = 14.
When N = 13, 1N + 49 = 62 and 4.5N = 58.5. So
when N = 13, 1N + 49 > 4.5N.
When N = 15, 1N + 49 = 64 and 4.5N = 67.5. So when N = 15, 1N + 49 < 67.5.
The solutions for 1N + 49 < 4.5N are all whole number values of N greater than 14. N > 14.
Solving by using properties for inequalities.
1N + 49 < 4.5N. Subtracting 1N from each side, we have 49 < 3.5N. Dividing each side by 3.5 we have 14 < N or N > 14.
Caution: When you multiply or divide both sides by a negative the order is reversed. Changing the sign changes the order: 3< 5 but -3 > -5.
1N + 49 < 4.5N. Subtracting 4.5N from each side.
-3.5N + 49 < 0. Subtracting 49 from each side.
-3.5N < -49. Dividing both sides by -3.5, N > 14.
Curriculum decision: Properties of inequalities are not emphasized in Moving Straight Ahead.
Future Link In 8th grade algebra students solve inequalities for the dependent variable. And, in the Unit It’s in the System 8th grade students will learn the properties of inequalities, such as
C< 1.5N + 49.
Equivalent expressions
As we have seen, equivalent equations come into play as we rewrite an equation in order to solve it. Equivalent expressions also come into play.
Example: Concert tickets are $22, plus a $1.50 tax for each ticket, plus a one-time ordering fee of $3. The cost depends on the number of tickets ordered. If the bill is $285, how many tickets were bought?
The equation relating Cost and Number is C = 22N + 1.5N + 3.
In this case we have to solve 285 = 22N + 1.5N + 3.
This is equivalent to 285 = 23.5N + 3, or
282 = 23.5N, 12 = N, 22N + 1.5N, and 23.5N are all equivalent expressions.
This is an example of the Distributive Property. 22N + 1.5N = N(22 + 1.5) = 23.5N.
Note: 285 = 22N + 1.5N + 3 could also be solved from a table or graph of C = 22N + 1.5N + 3 by looking for (N, 285).
Learning Aids
- 1.2: Three Students Walking Rates
- 1.3: Three Students Pledge Plans
- 3.1: Pouches and Coins
- 4.1: Climbing Stairs
- 4.2: Constant of Proportionality
Teaching Aids
- 1.2: Walking Rate
- 1.3: Matching Representations
- 1.4A: Ms. Chang's Class Account
- 1.4B: Ms. Chang & Mr. Mamer
- 2.1A: Emile & Henri's Race
- 2.1B: Amit's Claims
- 2.2A: Introduction
- 2.2B: T-Shirts
- 2.3A: Alana's Pledge Plan
- 2.3B: New Pledge Plans
- 2.3C: Graph of y=5x-3
- 2.3D: Troy's Graphing Calculator
- 3.1A: Alana's Earnings
- 3.1B: Nicole's Strategy
- 3.3A: Set 3 Student Thinking
- 3.3B: Situation A
- 3.4: Two T-Shirt Plans
- 4.1: Stairs on a Graph
- 4.2: A Set of Stairs
Family Letter
Family Letter (PDF)
© 2022 Connected Mathematics Project at Michigan State University
Do not copy, share, or duplicate without permission.